Hello everyone!

Welcome back to Pythagoras Week.  If you missed it, be sure to check it out from the beginning.  Today, we're going back to ancient Greece to look at Euclid's proof of the Pythagorean Theorem.  It's not the most elegant proof, but it concludes Euclid's Elements, which is easily the most significant work in the history of math.

Once again, we begin with our right triangle.  This time, we'll draw a square on each of the three sides.  We'll also draw a few extra lines, as shown below.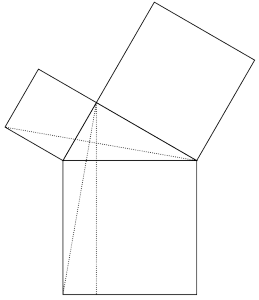The first thing to notice is that the two triangles shown in red below are the same (congruent), just rotated by .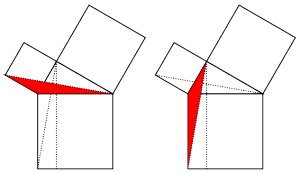The triangle in the left diagram above shares a base and height with the red square in the diagram below, and the triangle in the right diagram above shares a base and height with the red rectangle below.  The two triangles have the same area, so by the area formulas, the red square and rectangle have the same area.  By the same logic, the blue square and rectangle must also have the same area.  As in yesterday's proof, we get .

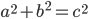There's a lot of interesting history surrounding this, and I'll be sure to go into detail at some point, but that's it for today.  See you again with a new post tomorrow.

by