Hello, everyone, and Happy Pi Day!
You've almost assuredly seen the number  before. It shows up in all sorts of odd places. So what is
before. It shows up in all sorts of odd places. So what is  ? You probably know
? You probably know  but that might feel like I'm pulling a number out of the air. Where does it really come from?
but that might feel like I'm pulling a number out of the air. Where does it really come from?
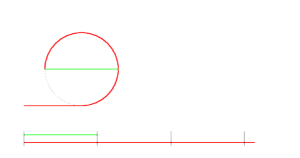
First, let's start with the definition:  is the ratio of a circle's circumference to its diameter. That is, if you took the circle and unrolled it (as below), the line segment you end up with is
is the ratio of a circle's circumference to its diameter. That is, if you took the circle and unrolled it (as below), the line segment you end up with is  times the width of the circle at its widest point.
times the width of the circle at its widest point.
 You've also probably heard that the area of a circle is
You've also probably heard that the area of a circle is 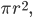 where
where  is the circle's radius. That's the same
is the circle's radius. That's the same  that we defined above. That's an odd coincidence; why might that be true? Well, lines and rectangles are a lot easier to work with than curvy things like circles (I'll come back to this when I rant about calculus), so let's make our circle look more like a rectangle. Slice the circle up into wedges, as below, and rearrange them as shown.
that we defined above. That's an odd coincidence; why might that be true? Well, lines and rectangles are a lot easier to work with than curvy things like circles (I'll come back to this when I rant about calculus), so let's make our circle look more like a rectangle. Slice the circle up into wedges, as below, and rearrange them as shown.
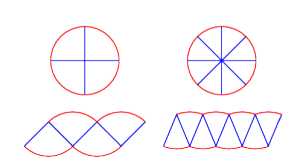 The resulting shape isn't quite a rectangle, as the top and bottom are bumpy and the sides are a bit slanted, but the more wedges we cut the circle into, the closer it becomes. In the limit, that is, as we pretend we can get infinitely many wedges, we actually get a rectangle. How big is it? The top and bottom are formed from the circumference of the circle, so the length of each is half the circumference. The sides each come from the radius of the circle. So the rectangle is
The resulting shape isn't quite a rectangle, as the top and bottom are bumpy and the sides are a bit slanted, but the more wedges we cut the circle into, the closer it becomes. In the limit, that is, as we pretend we can get infinitely many wedges, we actually get a rectangle. How big is it? The top and bottom are formed from the circumference of the circle, so the length of each is half the circumference. The sides each come from the radius of the circle. So the rectangle is  by
by  , and its total area is
, and its total area is 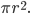
Now, I haven't exactly been rigorous here, but I hope I've at least given you some sense of why the circumference and area are so nicely related.
You might also wonder how we get the value 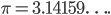 There are a lot of good ways to compute it. The ancient Greeks and Chinese found
There are a lot of good ways to compute it. The ancient Greeks and Chinese found  by considering inscribed polygons. Later mathematicians showed
by considering inscribed polygons. Later mathematicians showed  was equal to many infinite series. One of my favorite methods is known as Buffon's Needle.
was equal to many infinite series. One of my favorite methods is known as Buffon's Needle.
But for today, I'd like to show you a method based on throwing darts. Imagine you have a square dartboard with side length 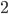 , and in it you draw a circle, as shown below. If you throw a dart at the board (without aiming), how likely is it to land in the circle?
, and in it you draw a circle, as shown below. If you throw a dart at the board (without aiming), how likely is it to land in the circle?
 The square has area
The square has area 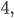 and the circle has area
and the circle has area  so you should hit it roughly
so you should hit it roughly  times in every
times in every 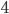 throws. I don't have the patience to throw that many darts, so I had my computer pick
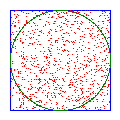
throws. I don't have the patience to throw that many darts, so I had my computer pick  random points on the dartboard.
random points on the dartboard. 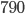 of them hit the circle, as shown below, giving me an estimate of
of them hit the circle, as shown below, giving me an estimate of  Trying again with
Trying again with 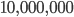 points (not shown), I got an estimate of
points (not shown), I got an estimate of 
 There's a whole lot more that could be said about
There's a whole lot more that could be said about  but I feel like I'm getting a bit long-winded, so I'll wrap it up here for today. Have a great Pi Day!
but I feel like I'm getting a bit long-winded, so I'll wrap it up here for today. Have a great Pi Day!